#271 - Domino Shading Puzzle-Pack
Here's a pack of five domino-shading puzzles that I made over the past few days. I hope you enjoy!
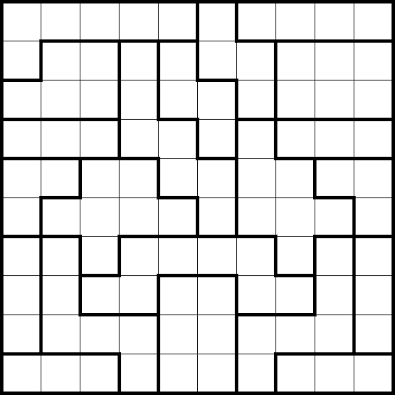
Norinori:
Shade some dominoes of cells so that every region contains exactly two shaded cells. Shaded dominoes may not touch orthogonally.
Norikabe:
Shade some dominoes of cells, dividing the grid into regions. Shaded dominoes may not touch orthogonally. Clues cannot be shaded, and every orthogonally connected area of unshaded cells contains exactly one clue, the value of which represents the size of the area.
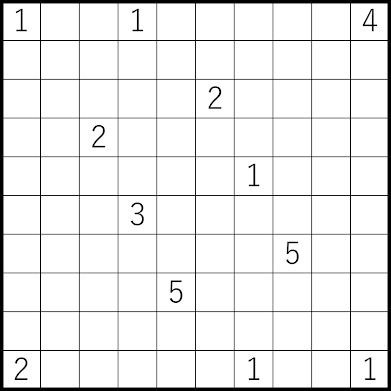
Double Yajisan-Kazusan:
Shade some dominoes of cells so that no two shaded dominoes are orthogonally adjacent and the remaining unshaded cells form one orthogonally connected area. If a cell with a number in it is unshaded, the number represents how many shaded dominoes can be seen in a straight line in the indicated direction, partially or totally. If a cell with a number in it is shaded, the number is meaningless.
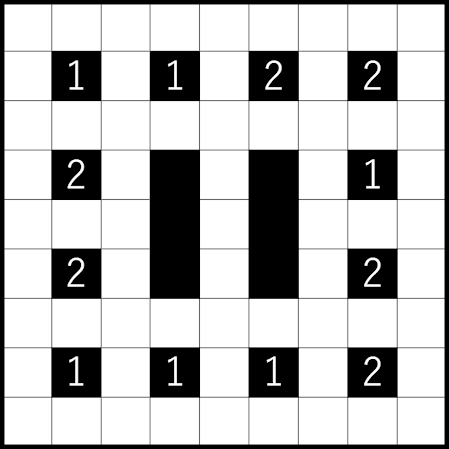
Dominion:
Shade some dominoes of cells to divide the grid into unshaded areas. Shaded dominoes may not touch orthogonally. Clues cannot be shaded, and each orthogonally connected area of unshaded cells contains exactly one type of clue, and all instances of it.
Double Kurodoko:
Shade some dominoes of cells so that no two shaded dominoes are orthogonally adjacent and the remaining unshaded cells form one orthogonally connected area. Clues cannot be shaded, and represent the total number of unshaded cells that can be seen in a straight line vertically or horizontally, including itself.







Comments
Post a Comment